엔지니어가 되고 싶은 공돌이
06. 평면벡터의 내적(Dot Product of Plane Vector) 본문
06. 평면벡터의 내적(Dot Product of Plane Vector)
Geca 2024. 4. 10. 12:22
6. 1. 평면벡터의 내적(Dot Product of Plane Vector)

- vector a, vector b의 내적(Dot Product)은 a · b = | a | | b | cos θ. [0° ≤ θ ≤ 180°]
- a · a = | a |2 .
- 내적은 결과는 스칼라(실수, Scalar, Real number)이다.
- a = (a1 , a2), b = (b1 , b2) => a · b = a1b1 + a2b2 .
- vector a, vector b, vector c에 대하여
1) 교환법칙(Commutative Property): a · b = b · a .
2) 결합법칙(Associative Property): (ka) · b = a · (kb) = k(a · b). [ k is real number]
3) 분배법칙(Distributive Property): a · (b + c) = a · b + a · c.
- cos θ = a · b / | a | | b | .
- | a ± b |2 = | a |2 ± 2a · b + | b |2. [a, b is vector]
6. 2. 평면벡터의 수직과 평행(Parallel and Perpendicular of Plane Vector)
- plane vector a, b 에 대하여
1) a ⊥ b <=> a · b = 0.
2) a // b <=> a · b = ± | a | | b |.
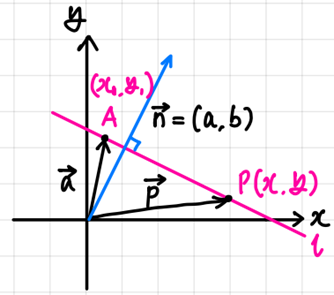
6. 3. 직선의 방정식(Equation of a Straight Line)
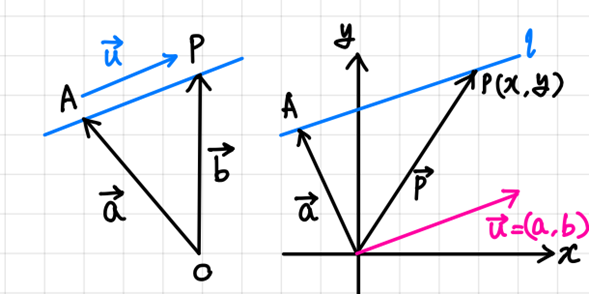
- position vector a 인 점 A를 지나고 vector u에 평행한 직선의 방정식
p = a + tu. [p is vector & t is real number]
- 점 A(x1 , y1) 을 지나고 vector u = (a, b) 에 평행한 직선의 방정식
(x – x1) / a = (y – y1) / b.
- 두 점 A(x1 , y1), B(x2 , y2) 를 지나는 직선의 방정식
(x – x1) / (x2 – x1) = (y – y1) / (y2 – y1).
- 점 A(x1 , y1) 을 지나고 vector n = (a, b) 에 수직인 직선의 방정식
a(x – x1) + b(y – y1) = 0.
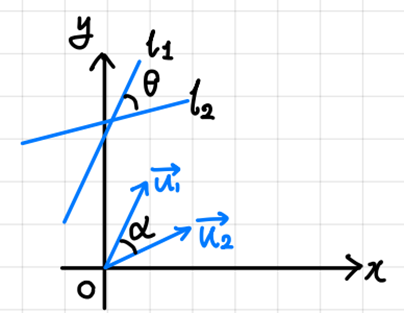
6. 4. 두 직선 각의 크기(Angle Between Two Lines)
- 두 직선 l1 , l2 의 vector가 각각 u1 = (a1 , b1), u2 = (a2 , b2) 일 때, 두 직선이 이루는 각의 크기를 θ[0 ≤ θ ≤ π/2] 라 하면
=> cos θ = | u1 · u2 | / | u1 | | u2 |.
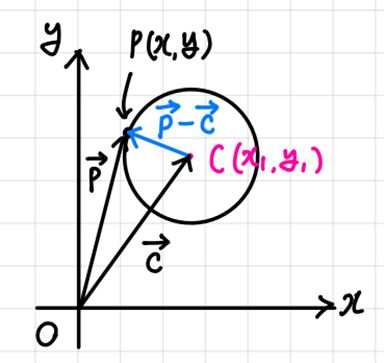
6. 5. 원의 방정식(Equation of a Circle)
- 점 C(x1, y1)을 중심으로 하고, 반지름의 길이가 r인 원의 방정식은
(x – x1)2 + (y – y1)2 = r2.
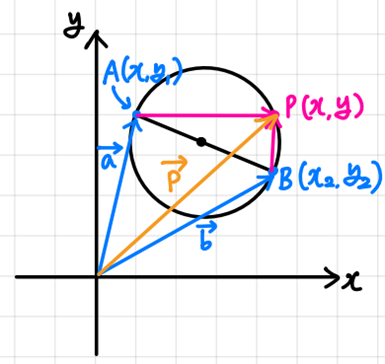
- 점 A(x1, y1), B(x2, y2)를 지름의 양 끝점으로 하는 원의 방정식은
(x – x1)(x – x2) + (y – y1)(y – y2) = 0.
'Basic Mathematics > High School_Geometry and Vector' 카테고리의 다른 글
| 08. 공간 도형(Three-dimensional Figure) (0) | 2024.04.12 |
|---|---|
| 07. 평면 운동(Plane Motion) (0) | 2024.04.11 |
| 05. 평면벡터의 성분(Components of Plane Vector) (0) | 2024.04.09 |
| 04. 평면벡터의 연산(Operation of Plane Vectors) (0) | 2024.04.08 |
| 03. 평면곡선의 접선(Tangent of Plane Curve) (0) | 2024.04.07 |